Unlocking Solutions: Mastering Quadratic Equations
Have you ever encountered a problem that seemed to have a curveball built into it? That's the essence of quadratic equations – they represent relationships where variables can have a squared impact. Think of a ball thrown in the air; its path isn't a straight line but a graceful arc. Describing that arc mathematically involves quadratic equations.
Quadratic equations, often presented in PDF documents for clear explanations and examples, are fundamental concepts in algebra. These equations involve a variable raised to the second power, leading to potential solutions, or "roots," where the equation holds true. Their significance goes beyond theoretical mathematics, finding applications in diverse fields like physics, engineering, computer graphics, and even financial modeling.
The history of these equations can be traced back centuries, with ancient Babylonian mathematicians developing methods to solve them for practical purposes, such as land surveying and inheritance division. These early mathematicians didn't have our modern notation, but they understood the underlying principles, laying the groundwork for further advancements.
The importance of quadratic equations lies in their ability to model a wide range of real-world phenomena. For instance, understanding their solutions helps us determine the trajectory of projectiles, optimize areas, analyze financial data, and even design structures. However, one of the main issues people face with quadratic equations is often rooted in their complexity. Grasping the different solution methods, such as factoring, completing the square, or using the quadratic formula, can be challenging, especially for those new to algebraic concepts.
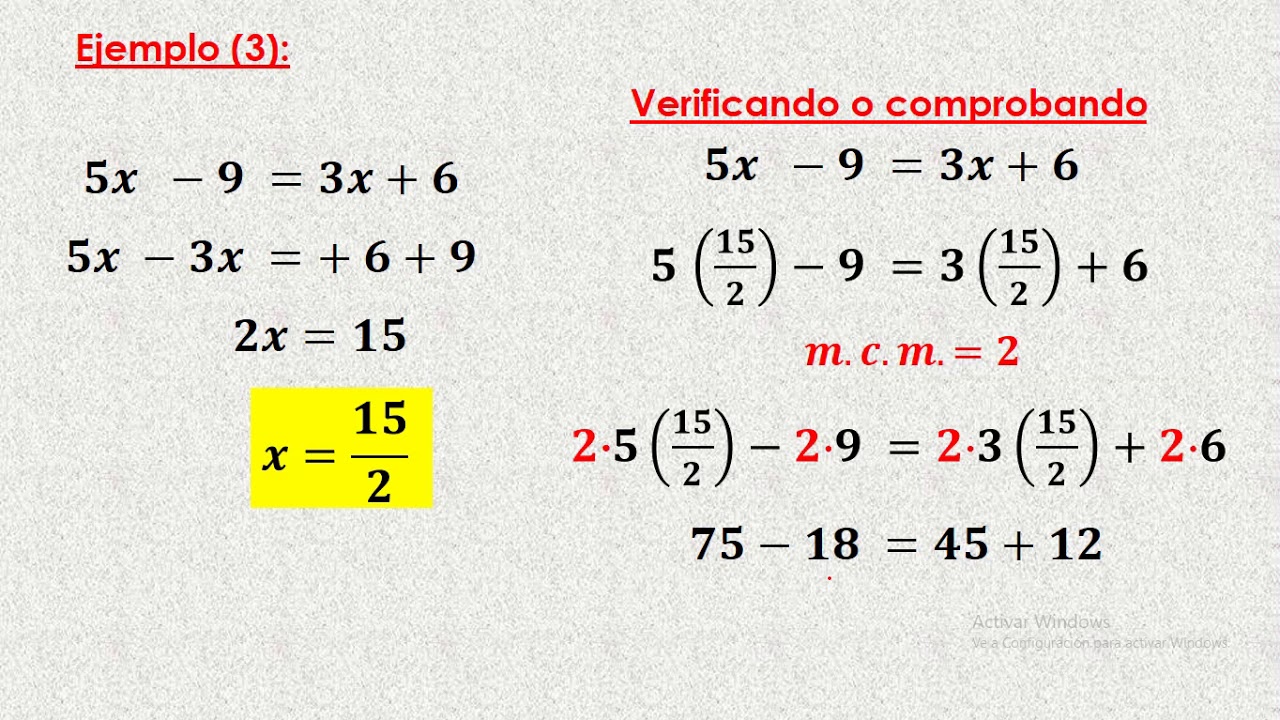
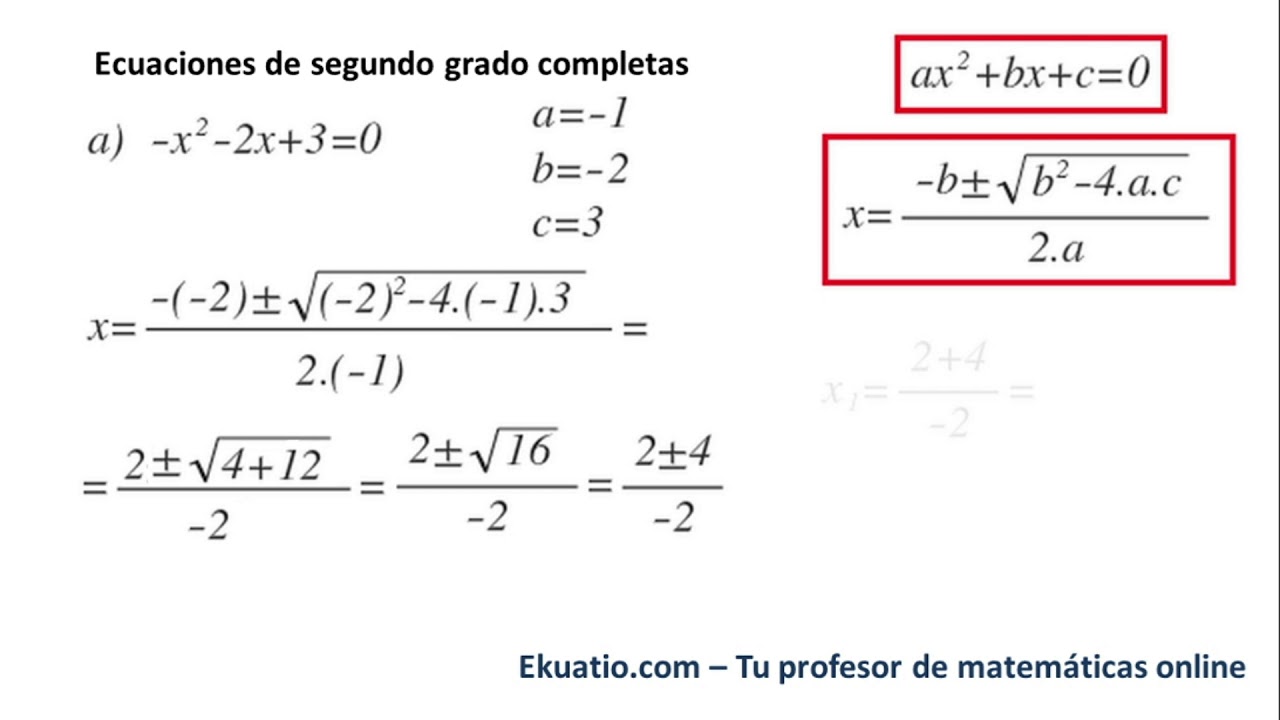
Let's break down the core of a quadratic equation. In its standard form, it is represented as ax² + bx + c = 0, where 'a,' 'b,' and 'c' are constants, and 'x' is the variable we aim to solve for. The power of '2' associated with 'x' is what makes it quadratic. Understanding this fundamental form is key to applying the right method for finding the solutions.
Advantages and Disadvantages of Learning Quadratic Equations
| Advantages | Disadvantages |
|---|---|
| Essential for understanding various scientific and mathematical concepts. | Can be initially challenging to grasp for some individuals. |
| Applicable in diverse fields, providing practical problem-solving skills. | Requires practice and familiarity with algebraic manipulations. |
| Enhances logical thinking and analytical skills. | Certain complex quadratic equations might require advanced techniques. |
To illustrate, imagine you're designing a fountain and want to determine the water's arc. By using a quadratic equation that represents the relationship between the water's height and horizontal distance, you can precisely calculate the fountain's shape. Similarly, in finance, quadratic equations are applied in risk assessment models and investment strategies.
As you delve deeper into the world of mathematics and its real-world applications, remember that mastering quadratic equations is a valuable asset. By embracing the challenge and seeking out resources, you equip yourself with a powerful tool for understanding and solving complex problems.
Taian mingyi machinery your solution for industrial equipment
Sculpt a stronger you effective back exercises for men at the gym
Finding comfort understanding oracion para los difuntos catolicos



.gif)