The Secret Life of 42: Unmasking Its Integer Divisors
Forty-two. It's the answer to the ultimate question of life, the universe, and everything, according to Douglas Adams. But what about the questions surrounding 42 itself? What makes this number tick? One way to understand a number is to break it down, to dissect it into its fundamental components. We're talking about its divisors, its factors – every single number that divides 42 cleanly. Let's unravel the mystery of all the factors of 42.
Understanding the divisors of a number is like understanding the ingredients of a cake. You wouldn't just shove a whole cake in your mouth without appreciating the flour, sugar, and butter, would you? Similarly, knowing all the factors of 42 gives us a deeper understanding of its mathematical nature.
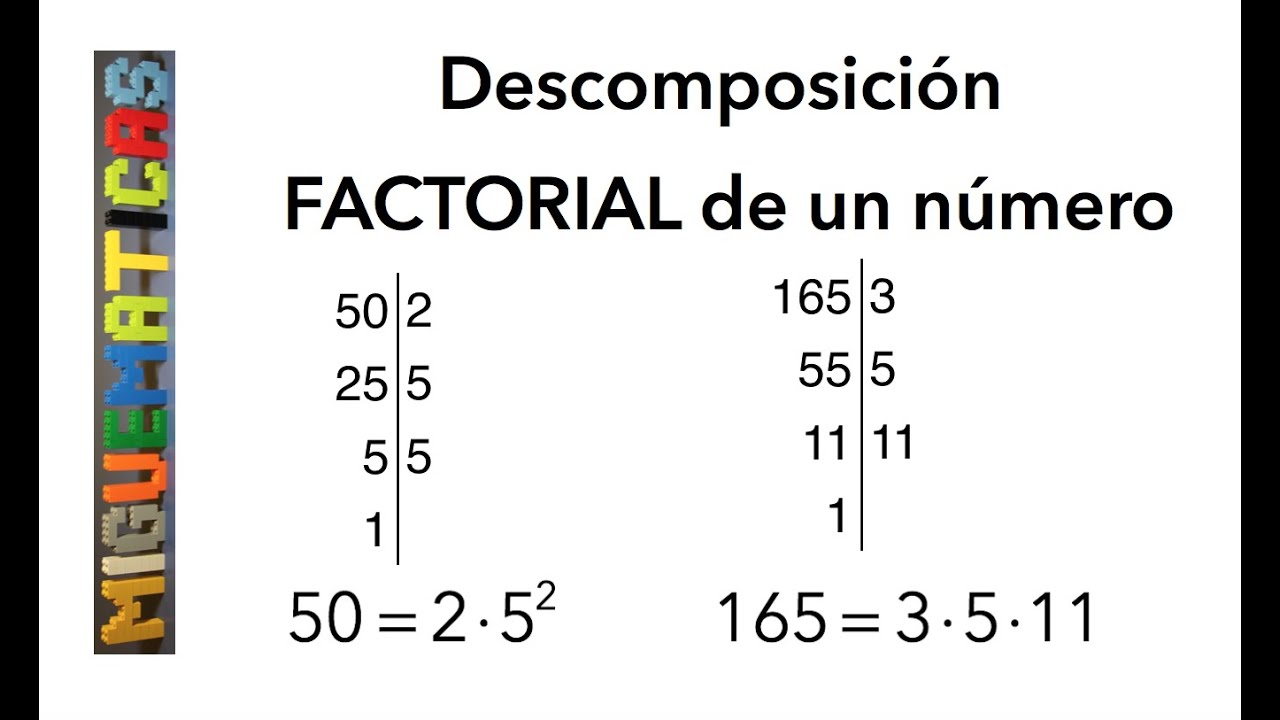
So, what exactly are the complete factors of 42? We're talking 1, 2, 3, 6, 7, 14, 21, and 42. These are the numbers that, when multiplied by another integer, result in 42. It’s a pretty exclusive club, and each member plays a vital role in the identity of 42. Imagine 42 as a building; these factors are the bricks that hold it all together. Knowing them allows us to build upon our understanding of more complex mathematical concepts.
The concept of factors dates back to the ancient Greeks, particularly with the work of Euclid. They were fascinated by numbers and their properties, and factors played a key role in their exploration of prime numbers and perfect numbers. Fast forward to today, and the concept of divisors is still crucial in areas like cryptography and computer science. Think of it like this: understanding the factors of 42 is a gateway to understanding more complex numerical relationships.
The importance of understanding the integer divisors of 42, or any number for that matter, can't be overstated. It forms the foundation for numerous mathematical concepts. From prime factorization to the least common multiple, factors are the building blocks of number theory. Imagine trying to build a house without knowing what bricks are. It's the same with math; you can't grasp more advanced concepts without understanding the basics, and factors are as basic as it gets.
Let’s define the factors of 42 a bit more formally: they are all the integers that divide 42 without leaving a remainder. For example, 42 divided by 7 is 6 with no remainder, so 7 is a factor. However, 42 divided by 5 is 8 with a remainder of 2, so 5 is not a factor of 42.
Here are three benefits of understanding the divisors of 42 (and other numbers): 1) Simplifying fractions: Knowing the factors helps in reducing fractions to their simplest form. 2) Finding the greatest common divisor (GCD): This is essential for various mathematical operations. 3) Understanding divisibility rules: This makes it easier to determine if a number is divisible by another.
Now, let's examine how knowing the factors of 42 can be practically applied. Suppose you have to divide 42 candies equally among a group of children. Knowing the factors (1, 2, 3, 6, 7, 14, 21, and 42) tells you how many children you can have in the group to ensure a fair distribution.
Advantages and Disadvantages of Focusing on the Factors of 42
Advantages: It strengthens fundamental number sense. It serves as a stepping stone to more complex mathematical concepts. It’s a great way to introduce basic number theory.
Disadvantages: Focusing solely on one number's factors might not be broadly applicable. It can be perceived as a trivial pursuit if not connected to broader mathematical principles.
Frequently Asked Questions:
1. What is a factor? A factor is a number that divides another number evenly.
2. How do you find the factors of a number? You can find factors by systematically dividing the number by integers starting from 1.
3. What are prime factors? Prime factors are factors that are also prime numbers.
4. What is the greatest common factor? The greatest common factor (GCF) is the largest number that divides two or more numbers evenly.
5. What is the least common multiple? The least common multiple (LCM) is the smallest number that is a multiple of two or more numbers.
6. Why is understanding factors important? Understanding factors is fundamental to many mathematical concepts.
7. How are factors used in real life? Factors are used in various situations like dividing items equally or simplifying fractions.
8. Are factors only positive numbers? Generally, we focus on positive factors, but numbers can also have negative factors.
Tips and tricks for finding factors: Start with 1 and the number itself. Then check divisibility by 2, 3, and so on, up to the square root of the number.
In conclusion, understanding all the factors of 42 – 1, 2, 3, 6, 7, 14, 21, and 42 – might seem like a small detail, a niche mathematical pursuit. But it’s much more than that. It's a window into the intricate world of numbers, a foundation for more complex concepts, and a testament to the elegance and order of mathematics. Knowing these divisors empowers us to simplify fractions, calculate the GCD and LCM, and appreciate the interconnectedness of mathematical principles. So, the next time you encounter the number 42, take a moment to acknowledge its constituent parts, its factors. They are the silent heroes, the unsung components that contribute to the richness and depth of this seemingly ordinary number. By understanding these fundamental elements, we unlock a deeper appreciation for the magic of mathematics and open doors to further exploration and discovery. Dive deeper into number theory and discover the fascinating secrets hidden within the world of numbers. You might be surprised at what you find!
Unlocking the power of stick figure art
Spice up your dd game dd magic items homebrew ideas that will blow your mind
Unlocking financial clarity your guide to monthly financial statements