Mastering Fraction Arithmetic: Addition and Subtraction
Ever struggled to divide a pizza equally among friends or calculate discounts at the grocery store? Understanding fractions is key to navigating these everyday scenarios and much more. This comprehensive guide will delve into the world of fraction arithmetic, specifically focusing on addition and subtraction. Whether you're a student, a parent helping with homework, or simply looking to brush up on your math skills, you'll find valuable insights and practical tips here.
Working with fractions can seem daunting at first, but with the right approach, it becomes manageable and even enjoyable. We'll explore the fundamental principles behind adding and subtracting fractions, breaking down the process into easy-to-follow steps. From finding common denominators to simplifying your answers, we'll cover everything you need to know to confidently tackle fraction problems.
The concept of fractions has been around for centuries, dating back to ancient civilizations like the Egyptians and Babylonians. These cultures needed ways to represent parts of a whole, whether for dividing land, measuring ingredients, or tracking trade. Today, fractions are integral to various fields, including cooking, engineering, finance, and science. Their importance lies in their ability to represent precise quantities and proportions.
One of the main challenges people encounter with fraction arithmetic is finding the least common denominator (LCD). This crucial step ensures that fractions are expressed in comparable terms, allowing for accurate addition and subtraction. We'll explore different methods for finding the LCD, including prime factorization and listing multiples. Another common issue is simplifying fractions to their lowest terms. This involves dividing both the numerator and denominator by their greatest common factor (GCF).
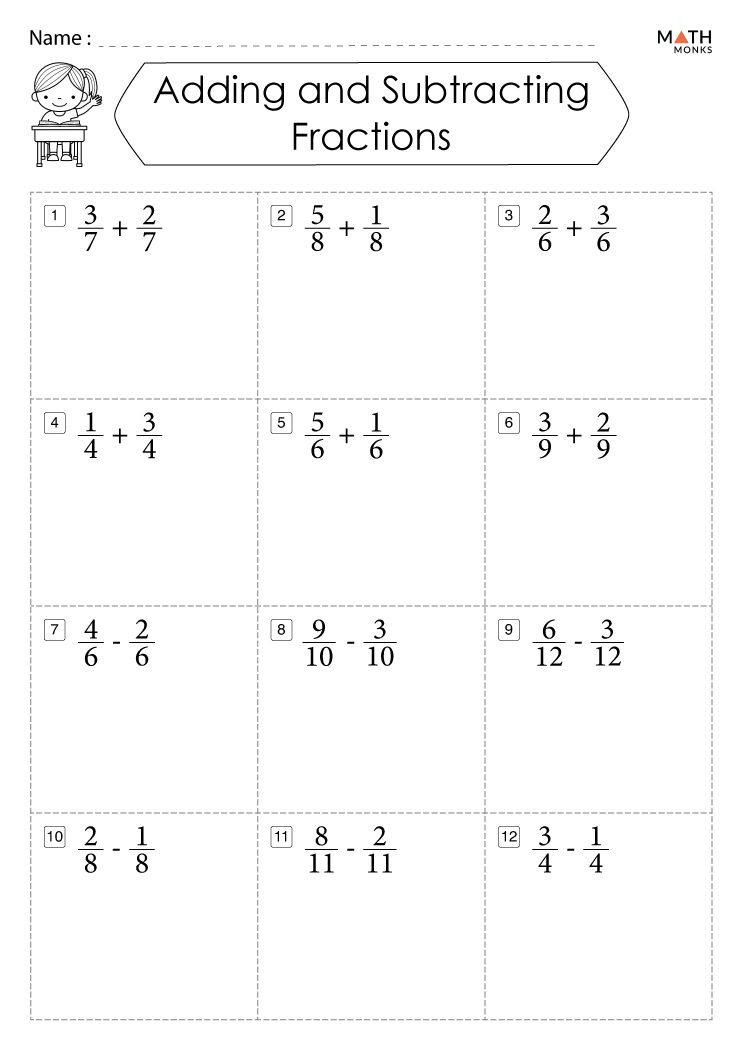
Adding and subtracting fractions with different denominators requires finding a common denominator. This common denominator allows us to express the fractions in equivalent forms, making the addition or subtraction possible. For example, if we want to add 1/2 and 1/3, we need to find a common denominator. The least common multiple of 2 and 3 is 6. We convert 1/2 to 3/6 and 1/3 to 2/6. Now we can add the numerators: 3/6 + 2/6 = 5/6.
One benefit of mastering fraction arithmetic is improved problem-solving skills. Working with fractions strengthens logical thinking and analytical abilities. For example, calculating proportions for recipes or determining discounts requires a solid understanding of fractions.
Another benefit is increased confidence in everyday math. Being comfortable with fractions simplifies tasks like splitting bills, measuring ingredients, and understanding sales percentages. This confidence translates to greater independence and resourcefulness.
A third benefit is a stronger foundation for advanced math. Fractions are essential for algebra, calculus, and other higher-level math concepts. Mastering fraction arithmetic prepares students for future academic success.
Action Plan: 1. Review basic fraction concepts. 2. Practice finding common denominators. 3. Work through addition and subtraction problems with increasing difficulty. 4. Seek help when needed from teachers, tutors, or online resources.
Advantages and Disadvantages of Using Fraction Worksheets (PDF)
| Advantages | Disadvantages |
|---|---|
| Printable and portable | Can be repetitive |
| Provide structured practice | May not cater to all learning styles |
| Offer immediate feedback (with answer keys) | Limited interactivity compared to digital resources |
Frequently Asked Questions:
1. What is a fraction? A fraction represents a part of a whole.
2. What is a common denominator? A common denominator is a shared multiple of the denominators of two or more fractions.
3. How do I find the least common denominator? You can find the LCD by listing multiples or using prime factorization.
4. How do I simplify a fraction? Divide the numerator and denominator by their greatest common factor.
5. Why are fractions important? Fractions are essential for various real-world applications and higher-level math concepts.
6. Where can I find practice problems? You can find practice problems in textbooks, online resources, and downloadable worksheets.
7. What are some real-world examples of using fractions? Examples include cooking, measuring ingredients, calculating discounts, and splitting bills.
8. How can I improve my fraction skills? Practice regularly, seek help when needed, and utilize various resources like worksheets, online tutorials, and educational apps.
Tips and Tricks: When adding or subtracting mixed numbers, convert them to improper fractions first. Remember to simplify your answers to lowest terms.
In conclusion, mastering addition and subtraction of fractions is a fundamental skill with far-reaching benefits. From everyday calculations to advanced mathematical concepts, understanding fractions empowers us to navigate the world with greater confidence and precision. By reviewing the concepts, practicing regularly, and utilizing available resources, including helpful "addition and subtraction of fractions pdf" worksheets, anyone can conquer fraction arithmetic and unlock its full potential. Don't hesitate to explore additional online resources and practice problems to solidify your understanding and build a strong foundation for future mathematical endeavors. Take the time to master these essential skills, and you’ll open doors to greater academic and practical success.
The alchemy of the slow cooker 3 pound roast
Acc basketball this weekend your guide to catching all the action
Resurrecting retro sound your hdmi tv and rca speakers can be friends